Most of the machine parts during their exploitation life are exposed to time-varying loads. During such loading, localized plastic deformation may occur. This plastic deformation induces permanent damage to the component and a crack develops [11]. The crack grows progressively and leads to sudden rupture of parts. This phenomenon is called fatigue failure. The main cause of fatigue failures is the presence of defects on the workpiece surface, such as scratches, sharp edges, cracks, etc. They act as a stress concentrators. As a result, fatigue cracks initiate and develop in depth and shortly thereafter the part breaks down.
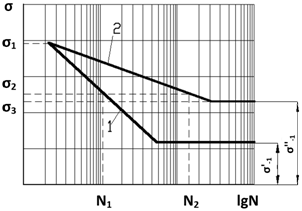 |
| Figure 1. Characteristic curve of fatigue [3]: 1 — before and 2- after application of the SPD process. |
Various studies show that there are indications that the finishing method, called surface plastic deformation (SPD) and its variant vibratory surface plastic deformation (VSPD), gives good results in increasing fatigue life for various metals and alloys [1,2,3,5]. For example, comparison of the fatigue curves before (curve 1) and after (curve 2) the application of SPD, are shown on Figure 1. It is seen that after SPD treatment, fatigue strength limit is significantly increased, compared to parts, threated with some of the existing traditional methods of finish machining (σ”-1 > σ’-1). The left side of the fatigue curve 2 has smaller slope than curve 1, which represents a specimen, not treated by SPD. Figure 1 shows that the number of cycles N2 to fatigue failure after implementation the SPD process is much greater than the number of cycles N1 (N2 >>N1).
The level of increase of the fatigue strength limit after the application of SPD depends on the design of the workpiece: the sharper the concentrators of stress are, the more efficient is the SPD process treatment. For example, in machine parts without a stress concentrator, the fatigue strength limit increases by 20-50%.
When workpieces have stress concentrators, the increase reaches up to 100 — 130% and even more. A similar effect of increasing the fatigue strength limit also has been seen after the application of the method vibratory SPD. Published studies [5] shows that when roller bearing type NU 2312 is used assembled directly on the steel shaft treated with vibro-SPD, the increase of the fatigue strength limit averaged in the range of 150-180%, and in some cases reaches 440%, in comparison with the polished shafts. This is explained with the optimization of the surface layer of the material by the formation of a completely regular roughness (RR) of the 4th type [2,4,5], which improves the conditions of contact as well as forms compressive residual stresses and increases the hardness in the surface layer of the material.
All of aforementioned studies are carried out, implementing SPD and vibro-SPD processes with kinematical schemes, using manually operated lathe and milling machines, combined with additional vibration generating devices. These additional devices are used for obtaining the necessary oscillating component of the movement of the ball tool to produce the needed intersection of toolpaths and to achieve RR roughness patterns of the 4th type [5]. These kinematical schemes of (V)SPD, using manually operated machines, have several significant drawbacks, as follows:
- a) Oscillations, driven by an external device during the process of vibro-SPD, causes dynamic stress of the technological system: machine tool — fixturing device — ball tool — workpiece, which may affect the stability of the process and the degree of uniformity of the cells of RR. Sometimes an intentional interruption of the contact between the ball tool and the surface, treated with vibro-SPD is necessary. These interruptions also lead to impact loads on the technological system.
- b) The speed of the spindle and the feed of the sliders in manually operated machines cannot be controlled infinitely adjustable, which limits the possibilities of control on the form and the dimensions of the cells from the resultant RR.
- c) The lack of precise movement synchronization between the various actuating mechanisms in the manually operated machine tools and these produced from additional vibratory devices often leads to variations of the dimensions and the shape uniformity of the cells of RR;
- d) The relatively smaller speeds and feeds of the traditional manually operated machine tools in comparison with the contemporary CNC machines produces relatively lower productivity of the SPD process in workpieces of larger sizes.
Due to these disadvantages of the conventional kinematical schemes to process functional surfaces by SPD or vibro-SPD, at the Technical University of Varna several new schemes for formation of the RR by SPD for planar and cylindrical surfaces, using the capabilities of modern CNC machine tools [10] have been developed. The ability to perform linear and circular planar and complex spatial interpolations makes CNC machines suitable to perform the needed complex toolpath. Together with appropriate CAD-CAM software for producing the needed NC code, they allow to achieve the complex movements of the ball tool without any need to use additional oscillations generating equipment. Here, the ball tool again follows needed complex trajectories (as well as in VSPD process), but in this case the toolpath generates using a mathematical model(s), which subsequently converted by CAM into corresponding NC program. This way the toolpath does not depend on the momentary combination of kinematical parameters of the process, running on the manually operated machines. These, in combination with the ability to infinitely adjustable change of the feeds in contemporary CNC machines, as well as the possibility to realize multi-axis schemes for processing complex shaped surfaces, opens up new opportunities for the application of the SPD process to increase the operational characteristics of a wide range of machine parts.
The technological scheme for processing by SPD, using CNC machines, kinematically differs from those, used in the past. Therefore, it is interesting to research the effects of the formed regular reliefs of the 4th type, obtained in various combinations of values of the regime parameters of the SPD process, using a new kinematical scheme onto the fatigue strength limits.
Therefore, the main purpose of the present work is to investigate the grade of significance of some important regime parameters of the SPD process, conducted under a new kinematical scheme over the number of cycles to failure of specimens, made of austenitic stainless steel grade 304L ASTM (Cr18Ni8). This steel exhibits an excellent resistance to a wide range of atmospheric, chemical, textile, petroleum and food industry exposures. Grade 304L is non-hardenable by heat treatment and have very good drawability. The combination of low yield strength and high elongation permits successful formation of complex shapes.
- Methodology
Due to the conducted preliminary studies of the SPD process, carried out using CNC machines [10], the significant regime parameters (see Fig. 2a), which mainly affect the characteristics of the resulting RR, have been established. They are the following:
- F, [N]– external force on the ball tool;
- i– number of the sinusoids within the length of the toolpath, determining the parameter , related to the height of the obtained RR cells;
- e, [mm]–half of the amplitude of the sine wave, related to the width of the obtained RR cells;
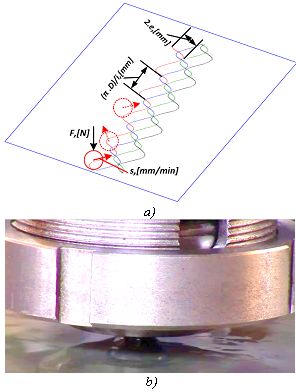
Figure 2. a) Scheme of the regime parameters of the SPD process, determining the shape and the dimensions of the RR cells of the 4-th type; b) Tool for SPD; |
|||||
| Table 1. Plan of the four factors full factorial design matrix with two levels per factor. | |||||
| № | Block | F, [N] | i | e, [mm] | s, [mm/min] |
| 1 | 1 | 1865 | 600.15 | 1 | 300 |
| 2 | 1 | 1865 | 600.15 | 2.5 | 150 |
| 3 | 1 | 1865 | 1200.15 | 1 | 150 |
| 4 | 1 | 1865 | 1200.15 | 2.5 | 300 |
| 5 | 1 | 3040 | 600.15 | 1 | 150 |
| 6 | 1 | 3040 | 600.15 | 2.5 | 300 |
| 7 | 1 | 3040 | 1200.15 | 1 | 300 |
| 8 | 1 | 3040 | 1200.15 | 2.5 | 150 |
| 9 | 2 | 1865 | 600.15 | 1 | 150 |
| 10 | 2 | 1865 | 600.15 | 2.5 | 300 |
| 11 | 2 | 1865 | 1200.15 | 1 | 300 |
| 12 | 2 | 1865 | 1200.15 | 2.5 | 150 |
| 13 | 2 | 3040 | 600.15 | 1 | 300 |
| 14 | 2 | 3040 | 600.15 | 2.5 | 150 |
| 15 | 2 | 3040 | 1200.15 | 1 | 150 |
| 16 | 2 | 3040 | 1200.15 | 2.5 | 300 |
- s,[mm/min] – feeding speed of the ball tool following the sinusoidal trajectory,
provided by the CNC milling machine;
Four factors full factorial experiment design with two levels per factor of type 24 has been realized to determine the degree of influence of these four mine factors and the interactions between them. The plan of the experiment is shown in Table 1. The diameter of the ball tool (see Figure. 2 b) is dball = 14 [mm] in all combinations of the regime parameters in the experimental plan in Table 1.
 |
| Figure 3. Experimental setup resonance vibration fatigue testing. |
The following experimental setup was created (see Figure 3) for the resonance vibration fatigue testing:
1 – First a Laptop Computer with installed PcLab2000-LT program, controlling the digital signal generator for generating sinusoidal excitation signals to drive the electromagnetic shaker;
2 — A Computer-controlled generator of wave sequences, type Velleman, model PCSGU250 to generate excitation signals, for the electromagnetic shaker;
3 — A linear amplifier to amplify the generated excitation signal to the required level to ensure stable working of the electromagnetic vibratory driver unit;
4 — An Electromagnetic shaker, type VEB RFT MESSELEKTRONIK “ÖTTO SHON” (DDR) with a fixture device for testing specimens and piezoelectric accelerometers, type KD 35-RTF;
5 — RFT 11032 type vibro-measuring devices, used to forward off the signals from the three piezoelectric accelerometers;
6 – A Bus-powered USB M Series multifunction data acquisition (DAQ) module, model NI USB-6216 (National Instruments), optimized for superior accuracy at fast sampling rates, that offers 16 analog inputs with 400 kS/s sampling rate;
7 — A 2nd Laptop Computer with an installation of LabVIEW System Design Software (National Instruments) running a specially designed algorithm for real-time monitoring of the test conditions. The algorithm can also record in log file a number of cycles to failure of each pair of specimens in accordance with the experimental plan.
2.1 Theoretical background.
For vibration fatigue test a beam-like specimen, mounted on an electromagnetic shaker, is used. This experimental setup can be modeled as a massless cantilever beam with lumped tip mass, shown on Figure 4. Detailed explanations of a dynamic model are given in [6].
An equivalent tip mass can be calculated from:
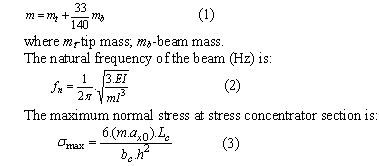
where ![]() — dynamic load amplitude; m-equivalent tip mass; bc,h, Lc – base, height of the concentrator cross section and length from the tip to the stress concentrator’s cross section.
— dynamic load amplitude; m-equivalent tip mass; bc,h, Lc – base, height of the concentrator cross section and length from the tip to the stress concentrator’s cross section.
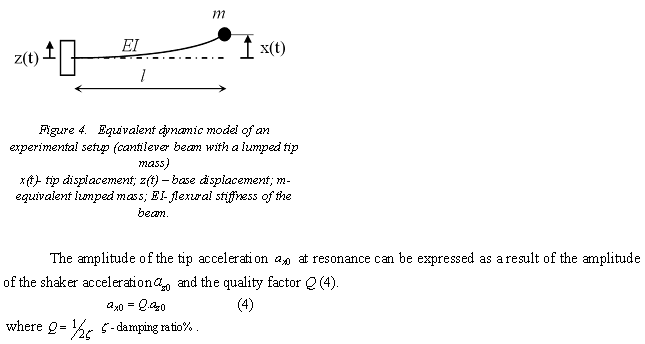
Formula (2) is used for approximate calculation of the length of the specimen, such as to fit its fundamental natural frequency in the range of 80-100Hz. From formula (3) stress concentrator dimensions can be fitted, such us the maximum stress to be below the yield limit of the material (Rp0.2).
2.2 Specimen design.
The used specimens are made of sheet stainless steel grade 304L ASTM (Cr18Ni8) with a thickness of 4 mm, which has physical and mechanical properties, as follows: ρ=8000kg/m3; E=190GPa, Rp0.2 = 324MPa, Rp1.0 = 360MPa, Rm=626MPa, A5=55%, A50= 52% and HB30 = 188.
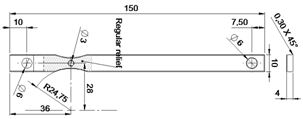 |
| Figure 5. Final design of fatigue specimen with an hourglass stress concentrator |
For preliminary design of the specimen, tip mass is measured (accelerometer +mounting stud). Choosing for the specimen width and length , equivalent mass and are calculated from (1) and (2). Fixing tip acceleration amplitude to and width of the concentrator section is needed to reach maximum stress amplitude about (3). The final design of the specimen is shown on Figure 5. As shown, an hourglass shape stress concentrator with a through hole with diameter of 3 mm in the narrowest section is designed to fit the chosen width.
2.3Numerical modeling
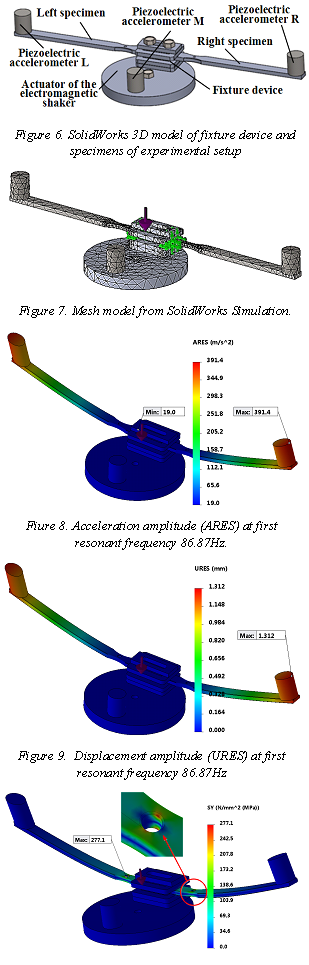
The final specimen design (see Figure 5) is verified by creating a 3D model of the vibrating set (Figure 3, position 4) in SolidWorks. The 3D-model is shown on Figure 6. To save time, two symmetrically mounted specimens with equal RR are simultaneously tested.
| Table 2. Natural frequencies and mass participation factors for the first 3 natural modes of vibration. | |||||
| Mode № | Frequency (Hz) | X direction | Y direction | Z direction | |
| 1 | 86.87 | 1.49.10-9 | 0.0564 | 6.991 | |
| 2 | 186.45 | 7.2074 | 0.1131 | 13.961 | |
| 3 | 765.02 | 14.134 | 1.2155 | 15.042 | |
In Simulation module of SolidWorks a linear modal analysis option is chosen to determine the natural modes of vibration and afterwards the displacements, stresses, strains, velocities, and accelerations of the specimen. Analysis parameters are set as follows:
- Mesh parameters (see Figure 7):
- Type: Curvature based mesh (which creates more elements in higher-curvature areas automatically)
- Maximum element size: 9.20 mm;
- Minimum element size: 1.84 mm;
- Min number of elements in a circle: 8;
- Element size growth ratio: 1.6;
- Jacobian points: 4;
- Material parameters (AISI 304):
- Elastic Modulus: 190000 N/mm2;
- Shear Modulus: 75000 N/mm2;
- Mass Density: 8000 kg/m3;
- Load parameters:
- Uniform Base Excitation: 19 m/sec2;
- Critical damping ratio for 1-15 mode: 0.03;
- Mass characteristics:
- Vibro meter sensor mass: 0.033 kg;
- Mass of the pair test elements: 0.091 kg.
The frequencies of the first tree natural modes are shown in Table 2. Distribution of acceleration, displacement and normal stress at the first resonance frequency are shown on Figures 8, 9, 10. As it can be seen from Figure 8 setting the acceleration amplitude of the shaker at 19 m/s2 gives a tip acceleration of 391.4m/s2, from which quality factor of the resonance Q=20.6 is determined. The maximum displacement amplitude at the tip is URES = ±1.312 mm, Figure.9 and the maximum normal stress SY=277.1Mpa is located at the stress concentrator cross-section, Figure 10. Comparing this stress with the Yield limit (Rp0.2 = 324MPa) of the material, it is clear that under these conditions, the current experimental study to determine the number of cycles to fatigue failure will be held in the zone of the elastic deformations.
2.4. Resonance fatigue testing procedure
 |
| Figure 11. Cut-off specimens with RR using a CNC laser cutting machine. |
The specimens are cut from a sheet with a thickness of 4 mm in the shape and the dimensions, shown on Figure 5, using a laser machine MAZAK SUPER X48. Before cutting in rectangular bands with a width of 10 and a length of 40 mm (where the stress concentrator section has to be) corresponding RR by SPD under a new scheme with a combination of regime parameters, according to the experimental plan from Table 1, on both sides of the plate is formed, Figure 11. After cutting, the sharp edges in the area of stress concentrators are tapered up to 0.3 mm by using fine sandpaper.
For every test, two specimens with an equal RR are mounted on the shaker. First, sweep sine driving signal at low amplitude isfed to the shaker to track resonance frequency. When the frequency is set, the driving signal amplitude is being adjusted till the tip acceleration becomes the same as the modeled one, Figure 8. Tip and base accelerations, as well as a number of cycles during the tests, are obtained and recorded, using a LabView routine. To highlight the effect of SPD under a new scheme and the availability of the RR on the fatigue life, additional specimens with the same shape and dimensions (see Figure 5), which have formed RR only on one side, and also such without RR, are tested.
- Statistical analysis of the results.
After conducting the tests, according to the methodology described in section 2, the results are obtained for the number of cycles to fatigue failure both the pairs specimens having RR with numbers from 1 to 16 from the planned four factorial experiment (see Table 1) and the reference specimens with unilaterally formed RR and those without any RR, which are shown summarized in Table 3. The specimens with unilaterally formed RR and those without any RR have been tested only for comparison purpose.
|
Table 3. Results obtained for the number of cycles to fatigue failure for tested specimens. |
||
| № (Numbers 1÷16 match those in the Table. 1) |
Specimen L(eft) | Specimen R(ight) |
| Both side RR -1 | 162938 | 159084 |
| Both side RR -2 | 70028 | 75028 |
| Both side RR -3 | 152720 | 174443 |
| Both side RR -4 | 114480 | 118981 |
| Both side RR -5 | 76996 | 78996 |
| Both side RR -6 | 64382 | 62331 |
| Both side RR -7 | 61275 | 63304 |
| Both side RR -8 | 254598 | 295546 |
| Both side RR -9 | 60944 | 62012 |
| Both side RR -10 | 23929 | 25158 |
| Both side RR -11 | 27921 | 29307 |
| Both side RR -12 | 48838 | 41046 |
| Both side RR -13 | 46880 | 45200 |
| Both side RR -14 | 300813 | 300813 |
| Both side RR -15 | 250202 | 247523 |
| Both side RR -16 | 26910 | 25512 |
| Without any RR — 1 | 18169 | 19304 |
| Without any RR- 2 | 18978 | 19032 |
| One side RR — 1 | 37302 | 35900 |
In conjunction with the intended objective of the present work, namely to investigate the grade of significance of some important regime parameters of the SPD process, conducted under a new kinematical scheme on the number of cycles to fatigue failure of specimens with bilateral RR, the results of experimental investigation (see Table 3) are subjected to a statistical processing, using standardized methods [8] in Mathcad (PTC). For brevity, the following symbols are accepted for the regime parameters of the SPD process, conducted under a new kinematical scheme: A= F, [N], B= i, C= e, [mm], D= s, [mm/min]. The possible two-factor interactions between the regime parameters are labeled as A.B, A.C, A.D, B.C, B.D, C.D. The results obtained at the 16 combinations of high and low levels of the regime parameters of the SPD process (see Table 3) are indicated with symbols Y1 ÷ Y16.
To build the so-called «The main effects diagram” the average effects Avglow and Avghigh are determined for the four main regime parameters and for the six interactions between them, using the following formulas:
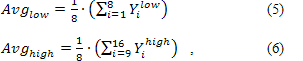
where: Yilow – are those eight results from Table 3, in which the regime parameters have low level; Yihigh – the other eight results from Table 3, in which the regime parameters have high level.
The differences between the values, obtained from the formulas (6) and (5), are calculated, using the formula:
![]() (7)
(7)
| Table 4. Effects of the main regime parameters of the SPD and their interactions. | |||
| Factor | Avglow | Avghigh | Eff |
| A | 84178.56 | 137580.06 | 53401.50 |
| B | 100970.75 | 120787.88 | 19817.13 |
| C | 106234.06 | 115524.56 | 9290.50 |
| D | 155659.13 | 66099.50 | -89559.63 |
| A.B | 105259.19 | 116499.44 | 11240.25 |
| A.C | 86741.50 | 135017.13 | 48275.63 |
| A.D | 154205.31 | 67553.31 | -86652.00 |
| B.C | 120573.56 | 101185.06 | -19388.50 |
| B.D | 128426.13 | 93332.50 | -35093.63 |
| C.D | 123913.69 | 97844.94 | -26068.75 |
| Blocks | 124070.63 | 97688.00 | -26382.63 |
 |
|||
| Figure 12. Graphics of the main effects of the four main regime parameters and the six interactions between them. | |||
Thus the corresponding magnitude of the effect of each the basic regime parameters of the SPD or interaction on the number of cycles to fatigue failure can be obtained.
With the values obtained for Eff in Table 4, the diagram of the main effects and interactions is prepared, which is shown in Figure 12. This graph clearly displays which regime parameters and their combinations are significant, thus allowing the assessment of the degree of their importance. As the differences Eff by absolute values (see Table 4) have a greater value, the length of the line in the graph is greater and consequently the effect of the associated regime parameter (or interaction between the regime parameters) of the SPD process is also larger, in terms of resultant number of cycles to failure. Furthermore, as can be seen from Figure 12, the effects can have either positive or negative values. When the effect of a regime parameter is positive, his graph is inclined to the right, suggesting that an increase in its value from low to high level increases the number of cycles to fatigue failure. Conversely, when the weighted effect of a regime parameter has a negative value, his graph is inclined to the left as it means that an increase in its value from low to high level reduces the number of cycles to fatigue failure.
The diagram in Figure 12 makes it clear that in this particular case the major factors A and D, and the interactions A.C and A.D have the greatest impact.
As a criterion for the selection of the significant regime parameters and interactions from those who are insignificant, the principle of «20-80» (also known as the Pareto Principle) is used. For that purpose from the Eff data in Table 4 by the formula:
![]() (8)
(8)
the absolute values of the half effects are calculated. In accordance with them the Pareto histogram and the cumulative curve are made, shown in Figure 13.
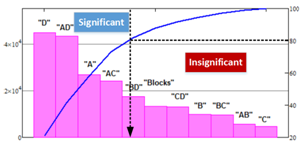 |
| Figure. 13. Histogram of arranging the regime parameters and their interactions by degree of significance. |
At level 80% on the right ordinate axis is drawn а horizontal line to the intersection with the Pareto cumulative curve (see Figure 13). Then in that cross point another vertical line is drawn. This vertical line divides the factors and their interactions (sorted in descending order by the degree of the effect over the number of cycles to failure) into two areas: those which are from the left side of the vertical line are considered «Significant» factors and those that remain on its right side are considered «Insignificant» factors. According to the bar chart of the main effects, shown in Figure 13, in this particular study two of the main factors D and A can be considered essential, as well as two of the factor interactions — A.D and A.C.
To verify the obtained results in Pareto analysis, two-way analysis of variance (ANOVA) are additionally carried out, and the obtained results are shown in Table 5. The labels of the columns of the Table 5 are as follows:
- SS — sum of squares between groups for each factor;
- DF — degrees of freedom;
- MS (mean squares) — SS divided by the degrees of freedom;
- F-statistic — MS divided by the MS error;
- P-value — probability of rejecting the factor, interaction, or blocking, based on its F-statistic when in fact it is significant.
At assigned confidence level α = 0.05 and identified degrees of freedom DFmin = 1 and DFmax = 31 (see Table 5) for the critical value of the criterion of Fisher the value Fcrit. = 4.16 is obtained. If the calculated value for the Fi for each of the four main factors and for the six possible interactions between them satisfies the expression Fi ≥ Fcrit., the hypothesis of the significance of a given factor or interaction is confirmed. Otherwise, when Fi ≤ Fcrit. the same hypothesis is rejected (i.e. factors or interactions are considered as insignificant).
Following this condition and comparing the calculated values for F from Table 5 with the Fcrit. it is clear that the hypothesis of significance reaffirms again for two main factors D and A, and for both interactions A.D and A.C (which are identified as significant after Pareto analysis) and for all other factors that hypothesis is rejected.
| Table 5. Results for factor significance after carried out two-way analysis of variance (ANOVA). | |||||
| Source | SS | DF | MS | F | P |
| A | 2.28E+10 | 1 | 2.28E+10 | 7.19 | 0.01 |
| B | 3.14E+09 | 1 | 3.14E+09 | 0.99 | 0.33 |
| C | 6.91E+08 | 1 | 6.91E+08 | 0.22 | 0.65 |
| D | 6.42E+10 | 1 | 6.42E+10 | 20.23 | 0.00 |
| A.B | 1.01E+09 | 1 | 1.01E+09 | 0.32 | 0.58 |
| A.C | 1.86E+10 | 1 | 1.86E+10 | 5.88 | 0.02 |
| A.D | 6.01E+10 | 1 | 6.01E+10 | 18.94 | 0.00 |
| B.C | 3.01E+09 | 1 | 3.01E+09 | 0.95 | 0.34 |
| B.D | 9.85E+09 | 1 | 9.85E+09 | 3.11 | 0.09 |
| C.D | 5.44E+09 | 1 | 5.44E+09 | 1.71 | 0.21 |
| Blocks | 2.78E+09 | 1 | 2.78E+09 | 0.88 | 0.36 |
| Error | 6.34E+10 | 20 | 3.17E+09 | — | — |
| Total | 2.55E+11 | 31 | — | — | — |
In order to examine the influence of the interactions A.C and A.D, the low and high values of factor A (i.e. F, [N]) are taken from Table 1 and the values of the factor D (i.e. s, [mm / min]) are calculated, based on the results from Table 3 and formulas (9) as follows:
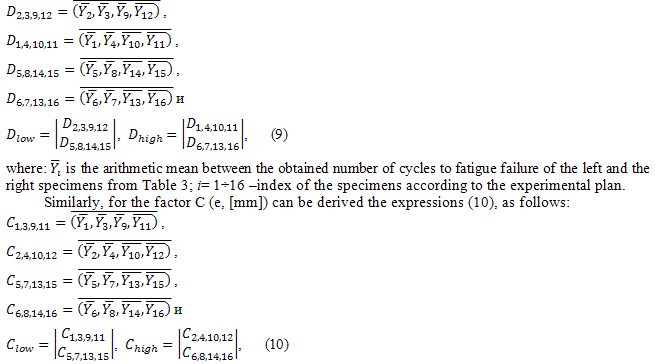
The graphs of the interactions obtained according to the expressions (9) and (10) are shown in Figure 14 a, b. They illustrate the effect of the interaction between the Low and the High levels of the interactions A.D and A.C. Through them it is possible to determine the influence of the regime parameters of the SPD process, conducted under a new kinematical scheme over the number of cycles to fatigue failure of the tested specimens.
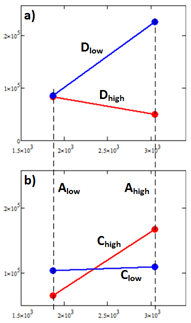 |
| Figure 14. Type of interactions : a) A.D (F. [N] — s,[mm/min]) and b) A.C ( F. [N] — е,[mm]). |
- Analysis and discussion.
Analyzing the graphs of the main effects, shown in Figures 14 a) and b) according to [7, 9], it becomes clear that in both cases there is a presence of a strong interaction between factors A and D and C and A, i.e. between the regime parameters: external compressive force of the ball tool F, [N] and the speed of the feed movement s, [mm / min] there is a strong interaction. The interaction between external compressive force of the ball tool F, [N] and the half of the amplitude of the sinusoidal trajectory of the ball tool e, [mm] is similar.
Figure 14 a) shows that at low values of F (Alow) the speed of the feed movement has a negligible impact on the number of cycles to fatigue failure, while at higher values of F (Ahigh) the impact is significant. In the lower value of s, up to 4,5 times more cycles to fatigue failure are obtained than at the higher value of s. Therefore, it can be concluded that the maximum effect on the number of cycles to fatigue failure is achieved when F → max and s → min.
Figure 14b) shows that with the increase of the value of the external force F from the low (Alow) to the higher value (Ahigh) the number of cycles to fatigue failure is also increased. At low value of the factor Clow (i.e. the regime parameter e, [mm] which is the half of the amplitude of the sinusoidal trajectory of the ball tool — see Fig. 2a), the increase of external compressive force from Alow to Ahigh, the increase in number of cycles to fatigue failure is insignificant in comparison with that which is obtained at the higher value of the Chigh. This tendency can be explained by the fact that in larger values of the parameter C, the ball tool runs on unit area of the treated surface by the SPD method more times than at lower values of C. The consideration of the averages of the participating regime parameters in this interaction – mean(A1, A2) and mean(C1, C2) once again confirms the hypothesis that the degree of influence of the parameter A is several times greater than the parameter C.
Based on the described methodology of the experimental studies and the obtained results the following findings and conclusions can be made:
- The presented methodology and the setting for the experimental investigation, based on forced vibrations in cantilevered beam at the first resonance frequency allows relatively fast determination of the number of cycles until the occurrence of fatigue failure of the experimental specimens. It allows simultaneously testing of up to two specimens, which halves the time for experimental research upon receipt of stable and repeated results.
- The obtained results (see Table 3) show that the number of cycles to fatigue failure in all specimens with bilateral formed RR by new kinematical scheme SPD process, exceed the total number of cycles, obtained for the specimens having unilaterally formed RR in the range from 100 to 800%. Compared to the specimens without any RR the exceedance is from 900% up to 14 times.
- Fatigue cracks in 100% of the tested specimens with unilateral RR occur and evolve from untreated by the SPD process side of the specimen, which highlights the beneficial influence of this treatment.
- Regarding the research on the degree of significance of the regime parameters of the SPD process, conducted under a new kinematical scheme, from Figure 12 it can be seen that the greatest effect on the number of cycles to fatigue failure is due to the speed of the feed movement of the ball tool s, [mm/min], and the next most significant factor is the external compressive force F, [N]. The interactions between parameters F and s, and between F and e appears to be significant. Furthermore, the conducted two-way analysis of the variance (ANOVA) (see Table 5) confirms that the identified by Pareto histogram main effects and interactions of the regime parameters (see Figure 13) are significant.
- The obtained results lead to the conclusion that the lack of significance of the regime parameters i and e, which are related to the shape and dimensions of the formed cells of the RR formed by the SPD process do not have a significant effect on the number of cycles to fatigue failure of the specimens. The observed beneficial effect is mainly due to the parameters F and s, related to the change of the microstructure and hardening of the surface layer of the surfaces, treated by the SPD process. For this reason, it is advisable to carry out further tests to determine the physical and mechanical changes in hardened surface layer of the 304L stainless steel in order to clarify the causes for the observed substantial increase in the number of cycles to fatigue failure after implementation of the SPD process, conducted under a new kinematical scheme.
References:
- Обработка деталей поверхностным пластическим деформированием : монография /Асланян И.Р., Бубнов А.С., Емельянов В.Н., Исаев А., Зайдес С.А., Копылов Ю.Р., Кропоткина Е.Ю., Лебский С.Л., Матлин М.М., Мозгунова А.И., Попов М.Е., Шустер Л.Ш.; под ред. С.А. Зайдеса; Иркутский гос. техн. ун-т, Российская инженерная академия. — Иркутск : Изд-во ИрГТУ, ISBN 978-5-8038-0869-5, 2014. — 559 с.;
- Б. В. Лупкин, Н. С. Погребельный, Упрочняющая обработка поверхностным пластическим деформированием, Cборник научных трудов, Выпуск 58, Харьков, «ХАИ» 2013, ISSN 2071-1077, 40-48 стр.
- Емельянов В. Н., Технология обработки деталей машин поверхностным пластическим деформированием : учебное пособие, Федеральное агентство по образованию, Новгородский гос. ун-т им. Ярослава Мудрого. — Великий Новгород, 2006, 139 с. ил.;
- Лебедев, В. А., Технология динамических методов поверхностного пластического деформирования, Федер. агентство по образованию, Дон. гос. техн. ун-т, Азов. технол. ин-т. — Ростов-на-Дону: ДГТУ, 2006, ISBN 5-7890-0357-5, 182 с.: ил.
- Шнейдер Ю.Г. Эксплуатационные свойства деталей с регулярным микрорельефом, изд. ИВА, Санкт-Петербург, 2001, ISBN 5-7577-0166-8, 261 с.;
- Dimitrov, D.M, Chirikov, V.A., Dimitrov, J., Analysis of fatigue life of thin plates from 40kh steel, Scientific proceedings from „NDT days 2015”, Year XXIII, Issue 2 (165), June 2015, ISSN 1310-3946. pp. 435-439 (in Bulgarian);
- Klaus Hinkelmann, Evaluating and Interpreting Interactions, Technical Report Number 04-5, Department of Statistics Virginia Tech Blacksburg, 2004, 30pp.; (9-10)
- Michael H. Kutner, John Neter, Christopher J. Nachtsheim, William Li, Applied Linear Statistical Models (5th ed.), McGraw-Hill Education, 2004, ISBN: 978-0071122214, 1396 pp. (827-829)
- Ranjit K Roy, A Primer on the Taguchi Method, Second Edition, SME, 2010, ISBN: 978-0872638648, 304pp; (58-60)
- Slavov S. D., “Opportunities for application of advanced CAD-CAM systems and CNC machines for precise processing of regular reliefs on external planar and cylindrical surfaces of machined parts”. International virtual journal «Machines, Technologies, Materials», Issue 8/2011, ISSN 1313-0226, pp 29-33;
- Yung-Li Lee, Jwo Pan, Richard B. Hathaway, Mark E. Barkey, Fatigue Testing and Analysis (Theory and Practice), Elsevier Inc, (2005) 402 pages.[schema type=»book» name=»EXPERIMENTAL RESEARCH OF THE EFFECT OF THE REGULAR SHAPED ROUGHNESS FORMATED BY USING NEW KINEMATICAL SCHEME FOR SURFACE PLASTIC DEFORMATION PROCESS ON THE NUMBER OF CYCLES TO FATIGUE FAILURE OF STAINLESS STEEL 304L (CR18NI8) » description=»This work presents the results of an experimental research, conducted to identify the level of the effects of certain regime parameters of the finishing process Surface Plastic Deformation (SPD), conducted under a new kinematic scheme, using machine tools with CNC control on the fatigue life of the specimens, made of stainless steel grade 304L ASTM (Cr18Ni8). The individual steps of the study methodology and the elements of the used experimental setup are described. The obtained results are reported and the Pareto and ANOVA analyses are performed to clear up the main effects of the tested regime parameters and the significant interaction between them. Based on the obtained results, some conclusions and recommendations, concerning the application of the SPD process, conducted under a new kinematical scheme, are made.» publisher=»Slavov Stoyan Dimitrov, Dimitrov Diyan Minkov» pubdate=»2016-12-18″ edition=»euroasia-science_28.04.2016_4(25)» ebook=»yes» ]

